U Chart with SigmaXL

What is a U Chart?

The U chart is a type of control chart used to monitor discrete (count) data where the sample size is greater than one, typically the average number of defects per unit.

Defect vs. Defective

Remember the difference between defect and defective?
A defect of a unit is the unit’s characteristic that does not meet the customers’ requirements.
A defective is a unit that is not acceptable to the customers.
One defective might have multiple defects. One unit might have multiple defects but still be usable to the customers.

This control chart monitors the average defects per unit. It plots the count of defects per unit of a subgroup as a data point. It considers the situation when the subgroup size of inspected units for which the defects would be counted is not constant. The underlying distribution of the U-chart is Poisson distribution.

U Chart Equations

Data Point:       

Center Line: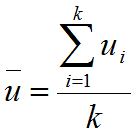     

Control Limits: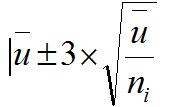

Where:

[unordered_list style=”star”]

• n_i is the subgroup size for the i^th subgroup
• k is the number of subgroups
• x_i is the number of defects in the i^th subgroup

[/unordered_list]

Use SigmaXL to Plot a U Chart

Data File: “U” tab in “Sample Data.xlsx”

Steps to plot a U chart in SigmaXL:

1. Select the entire range of the data
2. Click SigmaXL -> Control Charts -> Attribute Charts -> U
3. A new window named “U-Chart” appears with the selected range of the data automatically populated into the box below “Please select your data”.
   
4. Click “Next>>”
5. A new window named “U-Chart” pops up.
6. Select “Count of Defects” as the “Numeric Data Variable (Y)”
   Select “Count of Units Inspected” as the “Subgroup Column or Size”
7. Check the checkbox “Test for Special Causes”
   
8. Click “OK>>”
9. A new window named “Tests for Special Causes” pops up. Click “OK” to proceed.
   
10. The U chart appears in the newly generated tab “U-Chart (1)”.
    

U Chart Diagnosis

Model summary: Since the sample sizes are not constant over time, the control limits are adjusted to different values accordingly. The data point circled above falls beyond the upper control limit. We conclude that the process is out of control. Further investigation is needed to determine the special causes that triggered the unnatural pattern of the process.